- +61 7 3374 2877
- Email Us
This work explores the concept of interharmonics and subgrouping; a method introduced by the IEC 61000-4-7 [1] and IEC 61000-4-30 [2] standards to calculate both harmonics and interharmonics. Parameters that quantify the severity of distortion caused by interharmonics are defined and highlighted through examples.
An electrical signal can contain many frequencies. Some are integer multiplies of the fundamental (referred to as harmonics) and some are non-integer multiplies (referred to as interharmonics). The IEC 61000-4-30 standard defines an interharmonic frequency as “any frequency which is not an integer multiple of the fundamental frequency” [2], and includes frequencies lower than the fundamental, known as subharmonic frequencies.
Subgroups
The IEC 61000-4-7 [1] defines how instruments calculate the Root Mean Square (RMS) for both interharmonics and harmonics of a signal in a power system using the Discrete Fourier Transform (DFT); and requires a window size of 10 cycles for 50Hz and 12 cycles for 60Hz systems. The DFT produces a spectrum of frequency bands (bins) of 5Hz spacing as shown in Figure 1.
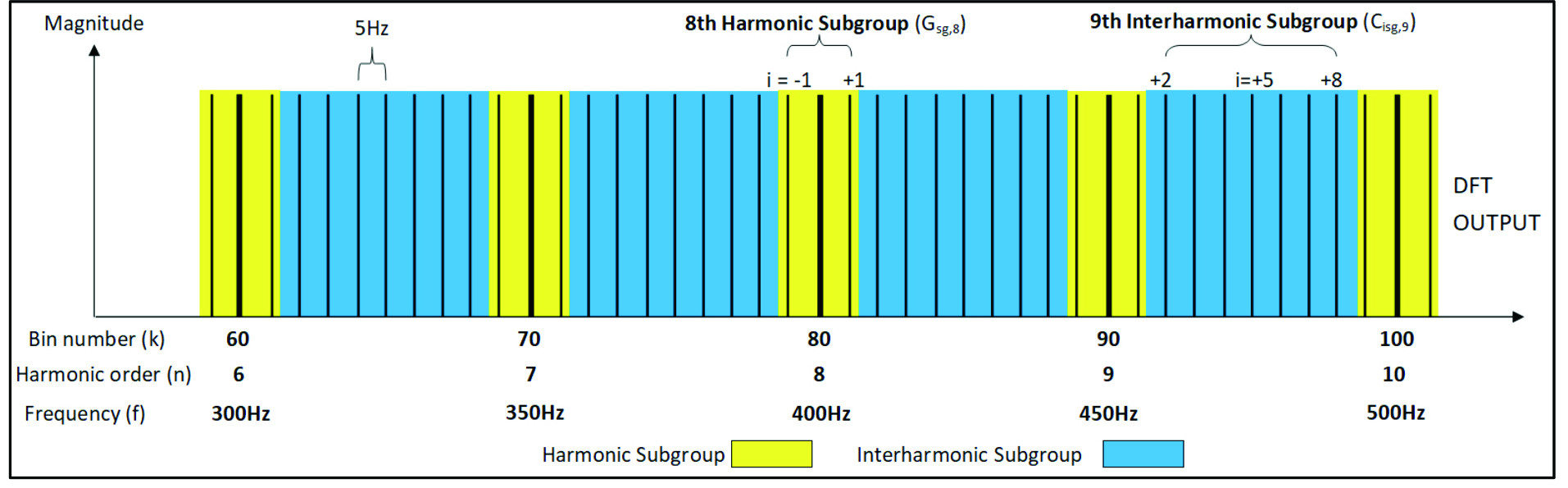
As shown in Figure 1, the harmonic bands are spaced 50Hz apart and highlighted in yellow; the interharmonic bands are highlighted in blue. Harmonic and interharmonic subgroups are made from frequency bands in Figure 1 and are calculated using Equations 1 and 2 as defined in IEC 61000-4-7 [1] and referred to by the IEC 61000-4-30 [2].
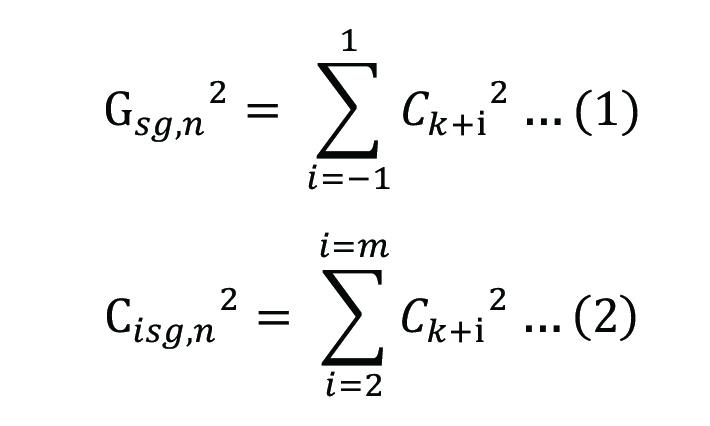
Where k is the bin number, Gsg,n and Cisg,n are the RMS values for the nth harmonic and interharmonic subgroups respectively. The variable m is equal to 8 and 10 for a 50Hz and 60Hz system respectively.